U1Ch1L4: Finding Patterns and Writing Protocols

Purpose: Students will begin to focus on representing numeric values using an infinite Binary Number System.

Challenge #1: How many Combinations Can Be Made From Just 2 Shapes?
Using the 2 shapes shown here, how many combinations can be assembles without repeating? In your Journals, begin assembling the combinations.

As an example, below is the first two combinations I came up with. Now it is up to you to discover the total number of combinations that can be assembled.

Total Number:                              

Challenge #2: How many Combinations Can be Made From These 3 Shapes?

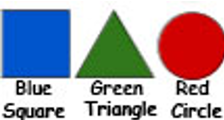
Using the 3 shapes shown hear, how many combinations can be assembles without repeating? Go to the Dry Erase Board and begin assembling combinations.

As an example, to the right is the first six combinations I came up with. Now it is up to you to discover the total number of combinations that can be assembled.

Total Number:                              

Challenge #3: Finding Patterns & Writing Protocols?
Step back and view the geometric shape combinations that you assembled. Was there a particular way that you organized it so that you could come to a final answer? Do you see any patterns with all the combinations? Can you rearrange the shapes to create patterns?
Write a protocol that will be handed to another group. When they follow your basic instructions, they should be able to easily create the pattern that you discovered. They will draw that pattern either in the space below or on the Dry Erase Board and have you check for accuracy.